The Riemann speculation is crucial open query in quantity idea—if not all of arithmetic. It has occupied consultants for greater than 160 years. And the issue appeared each in mathematician David Hilbert’s groundbreaking speech from 1900 and among the many “Millennium Issues” formulated a century later. The one who solves it would win a million-dollar prize.
However the Riemann speculation is a tricky nut to crack. Regardless of a long time of effort, the curiosity of many consultants and the money reward, there was little progress. Now mathematicians Larry Guth of the Massachusetts Institute of Know-how and James Maynard of the College of Oxford have posted a sensational new discovering on the preprint server arXiv.org. Within the paper, “the authors enhance a end result that appeared insurmountable for greater than 50 years,” says quantity theorist Valentin Blomer of the College of Bonn in Germany.
Different consultants agree. The work is “a outstanding breakthrough,” mathematician and Fields Medalist Terence Tao wrote on Mastodon, “although nonetheless very removed from totally resolving this conjecture.”
On supporting science journalism
When you’re having fun with this text, contemplate supporting our award-winning journalism by subscribing. By buying a subscription you might be serving to to make sure the way forward for impactful tales concerning the discoveries and concepts shaping our world in the present day.
The Riemann speculation issues the fundamental constructing blocks of pure numbers: prime numbers, values solely divisible by 1 and themselves. Examples embrace 2, 3, 5, 7, 11, 13, and so forth.
Each different quantity, akin to 15, might be clearly damaged down right into a product of prime numbers: 15 = 3 x 5. The issue is that the prime numbers don’t appear to comply with a easy sample and as a substitute seem randomly among the many pure numbers. Nineteenth-century German mathematician Bernhard Riemann proposed a strategy to take care of this peculiarity that explains how prime numbers are distributed on the quantity line—at the least from a statistical viewpoint.
A Periodic Desk for Numbers
Proving this conjecture would offer mathematicians with nothing lower than a sort of “periodic desk of numbers.” Simply as the fundamental constructing blocks of matter (akin to quarks, electrons and photons) assist us to grasp the universe and our world, prime numbers additionally play an essential position, not simply in quantity idea however in virtually all areas of arithmetic.
There are actually quite a few theorems primarily based on the Riemann conjecture. Proof of this conjecture would show many different theorems as properly—yet one more incentive to sort out this cussed downside.
Curiosity in prime numbers goes again 1000’s of years. Euclid proved as early as 300 B.C.E. that there are an infinite variety of prime numbers. And though curiosity in prime numbers persevered, it was not till the 18th century that any additional important findings have been made about these primary constructing blocks.
As a 15-year-old, physicist Carl Friedrich Gauss realized that the variety of prime numbers decreases alongside the quantity line. His so-called prime quantity theorem (not confirmed till 100 years later) states that roughly n/ln(n) prime numbers seem within the interval from 0 to n. In different phrases, the prime quantity theorem presents mathematicians a means of estimating the standard distribution of primes alongside a piece of the quantity line.
The precise variety of prime numbers could differ from the estimate given by the concept, nevertheless. For instance: In accordance with the prime quantity theorem, there are roughly 100/ln(100) ≈ 22 prime numbers within the interval between 1 and 100. However in actuality there are 25. There’s subsequently a deviation of three. That is the place the Riemann speculation is available in. This speculation provides mathematicians a strategy to estimate the deviation. Extra particularly, it states that this deviation can’t turn into arbitrarily giant however as a substitute should scale at most with the sq. root of n, the size of the interval into account.
The Riemann speculation subsequently doesn’t predict precisely the place prime numbers are positioned however posits that their look on the quantity line follows sure guidelines. In accordance with the Riemann speculation, the density of primes decreases in accordance with the prime quantity theorem, and the primes are evenly distributed in accordance with this density. Which means there are not any giant areas wherein there are not any prime numbers in any respect, whereas others are stuffed with them.
You can too think about this concept by interested by the distribution of molecules within the air of a room: the general density on the ground is considerably larger than on the ceiling, however the particles—following this density distribution—are nonetheless evenly scattered, and there’s no vacuum anyplace.
A Unusual Connection
Riemann formulated the conjecture named after him in 1859, in a slim, six-page publication (his solely contribution to the sector of quantity idea). At first look, nevertheless, his work has little to do with prime numbers.
He handled a selected operate, the so-called zeta operate ζ(s), an infinitely lengthy sum that provides the reciprocal values of pure numbers which are raised to the facility of s:

Even earlier than Riemann’s work, consultants knew that such zeta features are associated to prime numbers. Thus, the zeta operate can be expressed as a operate of all prime numbers p as follows:

Riemann acknowledged the complete significance of this reference to prime numbers when he used not solely actual values for s but additionally complicated numbers. These numbers include each an actual half and roots from adverse numbers, the so-called imaginary half.
You possibly can think about complicated numbers as a two-dimensional assemble. Slightly than mark some extent on the quantity line, they as a substitute lie on the aircraft. The x coordinate corresponds to the actual half and the y coordinate to the imaginary half:
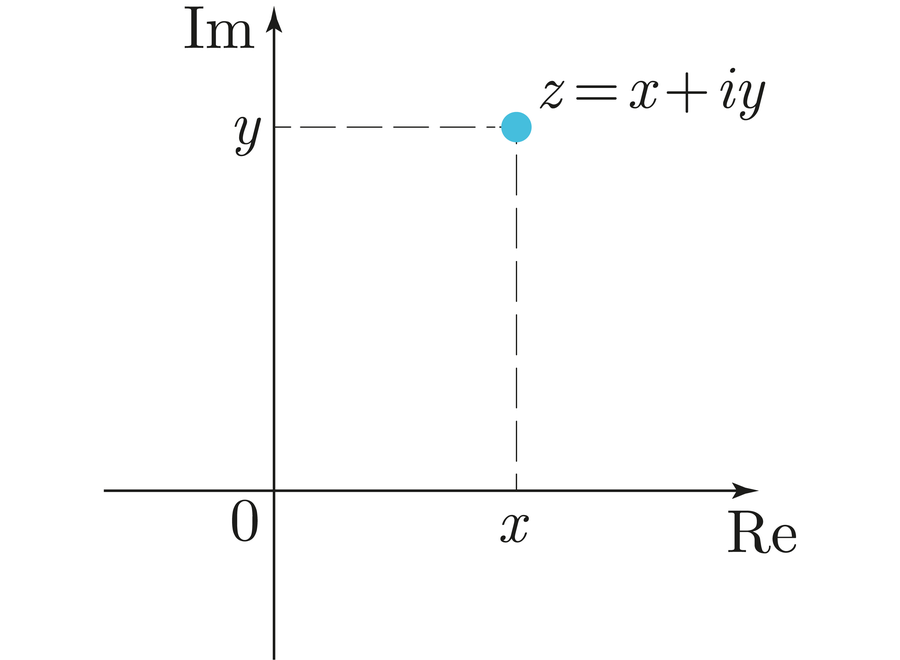
Никита Воробьев/Wikimedia
The complicated zeta operate that Riemann investigated might be visualized as a panorama above the aircraft. Because it seems, there are specific factors amid the mountains and valleys that play an essential position in relation to prime numbers. These are the factors at which the zeta operate turns into zero (so-called zeros), the place the panorama sinks to sea stage, so to talk.
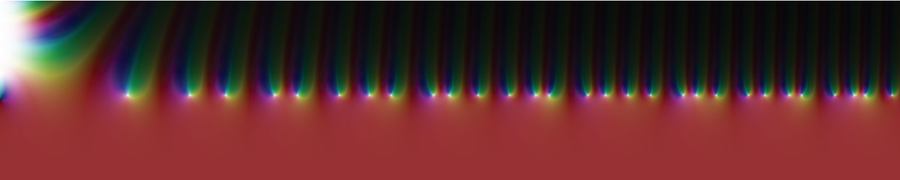
The colours signify the values of the complicated zeta operate, with the white dots indicating its zeros.
Riemann shortly discovered that the zeta operate has no zeros if the actual half is larger than 1. Which means the world of the panorama to the proper of the straight line x = 1 by no means sinks to sea stage. The zeros of the zeta operate are additionally recognized for adverse values of the actual half. They lie on the actual axis at x = –2, –4, –6, and so forth. However what actually Riemann—and all mathematicians since—have been the zeros of the zeta operate within the “crucial strip” between 0 ≤ x ≤ 1.
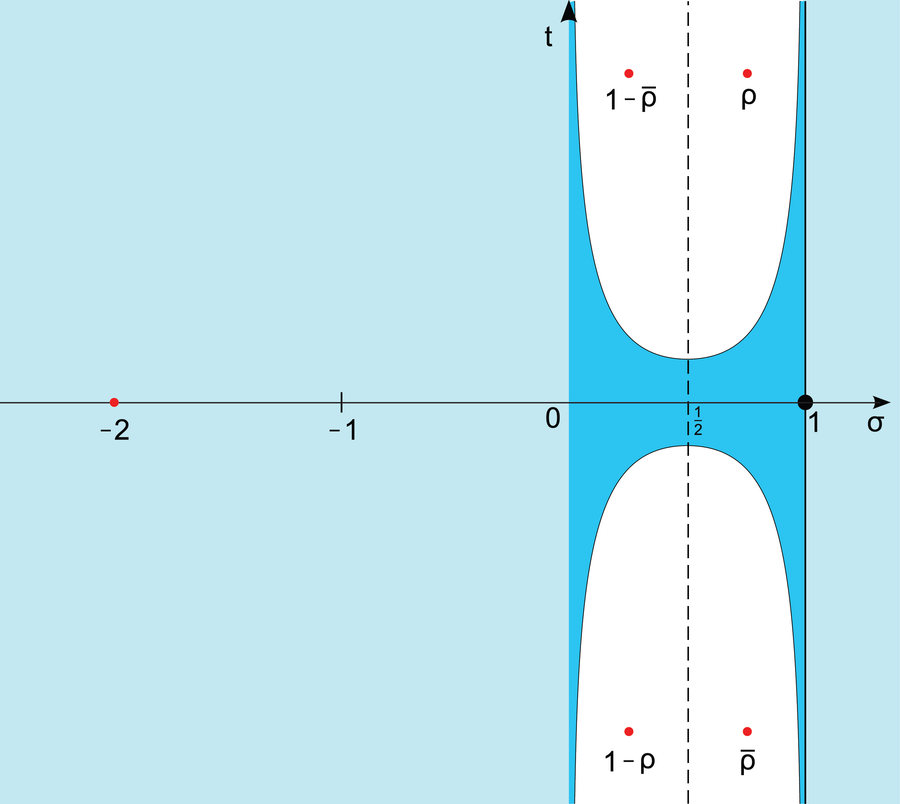
Within the crucial strip (darkish blue), the Riemann zeta operate can have “nontrivial” zeros. The Riemann conjecture states that these are positioned solely on the road x = 1/2 (dashed line).
Riemann knew that the zeta operate has an infinite variety of zeros throughout the crucial strip. However curiously, all seem to lie on the straight line x = 1/2. Thus Riemann hypothesized that each one zeros of the zeta operate throughout the crucial strip have an actual a part of x = 1/2. That assertion is definitely on the crux of understanding the distribution of prime numbers. If appropriate, then the position of prime numbers alongside the quantity line by no means deviates an excessive amount of from the prime quantity set.
On the Hunt for Zeros
Thus far, billions and billions of zeta operate zeros have now been examined—greater than 1013 of them—and all lie on the straight line x = 1/2.
However that alone is just not a sound proof. You’ll solely need to discover a single zero that deviates from this scheme to disprove the Riemann speculation. Subsequently we’re on the lookout for a proof that clearly demonstrates that there are not any zeros exterior x = 1/2 within the crucial strip.
So far, such a proof has been out of attain, so researchers took a special strategy. They tried to indicate that there’s, at most, a sure quantity N of zeros exterior this straight line x = 1/2. The hope is to scale back N till N = 0 sooner or later, thereby proving the Riemann conjecture. Sadly, this path additionally seems to be extraordinarily troublesome. In 1940 mathematician Albert Ingham was in a position to present that between 0.75 ≤ x ≤ 1 there are at most y3/5+c zeros with an imaginary a part of at most y, the place c is a continuing between 0 and 9.
Within the following 80 years, this estimation barely improved. The final notable progress got here from mathematician Martin Huxley in 1972. “This has restricted us from doing many issues in analytic quantity idea,” Tao wrote in his social media publish. For instance, should you wished to use the prime quantity theorem to brief intervals of the kind [x, x + xθ], you have been restricted by Ingham’s estimate to θ > 1/6.
But if Riemann’s conjecture is true, then the prime quantity theorem applies to any interval (or θ = 0), irrespective of how small (as a result of [x, x + xθ] = [x, x + 1] applies to θ = 0).
Now Maynard, who was awarded the distinguished Fields Medal in 2022, and Guth have succeeded in considerably enhancing Ingham’s estimate for the primary time. In accordance with their work, the zeta operate within the vary 0.75 ≤ x ≤ 1 has at most y(13/25)+c zeros with an imaginary a part of at most y. What does that imply precisely? Blomer explains: “The authors present in a quantitative sense that zeros of the Riemann zeta operate turn into rarer the additional away they’re from the crucial straight line. In different phrases, the more severe the doable violations of the Riemann conjecture are, the extra not often they might happen.”
“This propagates to many corresponding enhancements in analytic quantity idea,” Tao wrote. It makes it doable to scale back the dimensions of the intervals for which the prime quantity theorem applies. The concept is legitimate for [x, x + x2/15], so θ > 1/6 = 0.166… turns into θ > 2⁄15 = 0.133…
For this advance, Maynard and Guth initially used well-known strategies from Fourier evaluation for his or her end result. These are related strategies to what’s used to interrupt down a sound into its overtones. “The primary few steps are customary, and lots of analytic quantity theorists, together with myself, who’ve tried to interrupt the Ingham certain, will acknowledge them,” Tao defined. From there, nevertheless, Maynard and Guth “do a lot of intelligent and surprising maneuvers,” Tao wrote.
Blomer agrees. “The work supplies a complete new set of concepts that—because the authors rightly say—can in all probability be utilized to different issues. From a analysis viewpoint, that’s essentially the most decisive contribution of the work,” he says.
So even when Maynard and Guth haven’t solved Riemann’s conjecture, they’ve at the least supplied new meals for thought to sort out the 160-year-old puzzle. And who is aware of—maybe their efforts maintain the important thing to lastly cracking the conjecture.
This text initially appeared in Spektrum der Wissenschaft and was reproduced with permission.



